
Om Forum for
matematiske perler (og kuriositeter)
2001/2002 ·
2002/2003 ·
2003/2004 ·
2004/2005 ·
2005/2006 ·
2006/2007 ·
2007/2008 ·
2008/2009 ·
2009/2010 ·
2010/2011 ·
2011/2012 ·
2012/2013 ·
2013/2014 ·
2014/2015 ·
2015/2016 ·
2016/2017 ·
2017/2018 ·
2018/2019 ·
2019/2020 ·
2020/2021 ·
2021/2022 ·
2022→.
Neste foredrag blir annonsert her i tidens fylde …

Starting with the simplest number (which is …?), we survey the historical, logical and physiological foundations of numbers and sizes (cardinality, measures) as used and perceived by men and mathematicians. Some questions asked:
As indicated by the answers to these questions, some numbers do appear more natural than others.
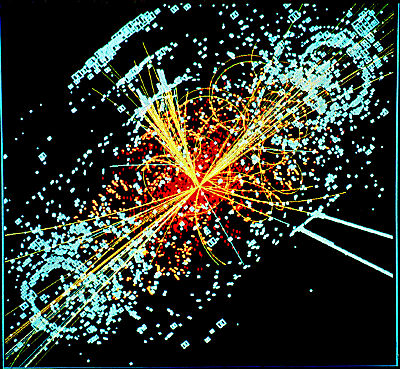
In the beginning of July 2012 CERN announced a press conference in Geneva to inform the world media about news from the LHC (Large Hadron Collider) accelerator concerning the possible discovery of a new particle. Professor Peter Higgs (aged 83) was invited from England, and he accepted the invitation. The news made its way to the front pages in newspapers all over the world, making big headlines everywhere.
The particle in question was the Higgs boson, theorized by professor Higgs in 1964. The media have made serious attempts to explain to the world what this particle is, and what it does. Friends of mine, who are mathematicians, have told me that there is much talk about the Higgs particle, gauge theory and so on, when they attend international conferences, but very few participants have real knowledge and/or understanding of what it is all about. The purpose of my lecture is to share with you some information that I have acquired with my background in particle physics, emphasizing the underlying mathematics like gauge theory and spontaneous symmetry breaking. Hopefully this will make it possible to give you an opportunity to grasp the essence of the physics involved as well.
Although the statement may appear cryptic at this point, I'll just mention briefly that the Higgs boson is a particle that influences the mass of other subatomic particles. It surrounds itself with a hitherto unknown field that interacts with such particles, and is itself the quantum of this field (like the photon is the quantum of the electromagnetic field.) The particle has been sought by experimentalists for more than 40 years, and no one can tell for certain whether the particle detected at LHC in spring 2012 really is the Higgs boson or not (it is a promising candidate though). The difficulty of detecting the Higgs particle has possibly to do with it being very exotic and scarce nowadays. It probably was plentiful and active early in the history of the universe, and did most of its job in the so-called condensation phase, when pure energy was transformed into matter (i.e. mass), and particles acquired different masses depending on the duration and intensity of their interaction with the Higgs boson and its surrounding (gauge-)field. The lecture will be concluded by retracing professor Higgs' steps when he made efforts to find equations (and Lagrangian densities) describing the mediators of weak interaction (responsible for the decay of free neutrons and the transition from muons to electrons).
The aim of the lecture is to show that the following cryptical passage from Gödel is a key to his philosophy of mathematics and that it makes good sense when we interpret it on the background of Husserl's philosophy, which he studied very thoroughly:
“That something besides the sensations actually is immediately given follows (independently of mathematics) from the fact that even our ideas referring to physical objects contain constituents qualitatively different from sensations or mere combinations of sensations, e.g., the idea of object itself ... . Evidently, the ‘given’ underlying mathematics is closely related to the abstract elements contained in our empirical ideas. It by no means follows, however, that the data of this second kind, because they cannot be associated with actions of certain things upon our sense organs, are something purely subjective, as Kant asserted. Rather they, too, may represent an aspect of objective reality, but, as opposed to the sensations, their presence may be due to another kind of relationship between ourselves and reality.”
Gödel, What is Cantor's continuum problem?, Supplement. Benacerraf, Paul & Putnam, Hilary (red.) Philosophy of Mathematics: Selected Readings. Englewood Cliffs, New Jersey: Prentice-Hall, 1964. p. 271–272. Trykket opp på ny i S. Feferman, J.W. Dawson, Jr., S.C. Kleene, G.H. Moore, R.M. Solovay & J. van Heijenoort (red.), Kurt Gödel. Collected Works, Vol. II, Publications 1938–1974. Oxford: Oxford University Press. 1990, p. 268.
[Every mathematician knows about Gödel, few know who Husserl is. He is known as the “father of phenomenology” in philosophy, but he started out as a mathematician writing his Ph.D thesis Beitrage zur Variationsrechnung under the supervision of Weierstrass and Königsberger (a former student of Weierstrass).]
One of the great classical results of complex analysis is Picard's theorem, stating that the image of an entire function can avoid at most one point in the plane.
As early as 1922, Fatou found a holomorphic map $\mathbb{C}^2\to \mathbb{C}^2$ whose image is not dense. An example of such a map that is one-one was found by Bieberbach in 1933.
The talk will start by looking at the dynamical behaviour of some analytic maps in one variable. In one variable, the dynamics of biholomorphic maps is not interesting, but we will see that this is no longer the case in higher dimensions. This will be a back-drop for how Rosay and Rudin (1988), as usual using simple techniques in a clever way, constructed many examples with the same properties as Fatou and Bieberbach got.
The remainder of the talk will focus on using elementary methods to create new mappings with other surprising behaviours.
Biology is becoming increasingly mathematized. But the intimate dialogue between mathematics and biology necessary to understand life has barely begun. For several hundred years physics has been the most influential branch of science for the development of mathematics. Considering that the living world dwarfs the inanimate world in terms of order and pattern to be explained, biology should not just be a major source of inspiration for development of new mathematics, it also implies that the orderliness of the living world is a major explanandum for an intellectual discipline claiming to be the science of pattern and order. The genotype-phenotype relation is at the core of theoretical biology. It will be argued why a mathematically based explanatory structure of this relation is in principle possible, and why it has to embrace both sequence to consequence and consequence to sequence phenomena. In this perspective the primary role of DNA in the chain of causality is that its presence allows a living system to induce perturbations of its own dynamics as a function of its own system state or phenome, i.e., it capacitates living systems to self-transcend beyond those morphogenetic limits that exist for non-living open physical systems in general. The talk may be considered a call to arms to mathematicians and the mathematically inclined to rise to the challenge of developing new formalisms capable of dealing with the deep defining characteristics of living systems.
The Riemann Hypothesis is one of the great unsolved problems in mathematics. It states that all the zeros of the zeta function $\zeta (s)$ are on the critical line $s = \frac{1}{2} + it$, $ -\infty \lt t \lt \infty $ (except the “trivial” zeros $-2$, $-4$, $-6$, … found by Euler). In my talk the influence of the zeros on the distribution of the prime numbers will be discussed. – However, a ferocious jungle of formulas and elaborate estimates cannot be spirited away! There is no elementary explanation to offer.
In his memoir Ueber die Anzahl der Primzahlen unter einer gegebenen Grösse Bernhard Riemann gave in 1859 an explicit formula[see below] for the number of primes less that a given bound. The formula runs over all zeros of the zeta function and, as fate would have it, the formula is optimal under the Riemann Hypothesis. The Hypothesis is equivalent to an absolute error term of the order $O(\sqrt{N} \log (N))$ in the Prime Number Theorem: $$\left| \pi (N) - \int^N_0 \frac{dy}{\log (y)} \right| \lt \frac{1}{8 \pi} \sqrt{N} \log (N),\qquad N \geq 2657$$ (von Koch 1901; Schoenfeld 1976), where $8 \pi$ is irrelevant and $\pi (N)$ = the number of primes $\leq N$. Without any hypothesis, no error term even of the form $O(N^{1-\epsilon})$ has been proved.
$$\begin{gather*} \pi (x) = \Pi (x) - \frac{1}{2} \Pi (x^{1/2}) - \frac{1}{3} \Pi (x^{1/3}) - \frac{1}{5} \Pi (x^{1/5}) + \frac{1}{6} \Pi (x^{1/6}) \cdots \\ \Pi_0 (x) = \int^x_0 \frac{dy}{\log (y)} - \sum^\infty_{k=1} \operatorname{Li} (x^{\rho_k}) - \log (2) + \int^\infty_{x} \frac{dy}{y(y^2 - 1) \log (y)} \\ \zeta(\rho_k) = 0,\quad \rho_k = \beta_k + i \gamma_k, x^{\rho_k} = x^{\beta_k} e^{i \gamma_k \ln (x)} \\ \Pi(x)=\Pi_0(x+0) \end{gather*}$$

The answer to this question is given by a beautiful theorem of Hardy and Ramanujan (1917), the theorem has a very nice quantification due to Erdős and Kac (1940). These results can be explained in a quite elementary way, by an elegant combination of ideas from number theory and probability theory. In my opinion both results deserve to be called “pearls” of the last century mathematics.
We will start with some elementary results in number theory, give a simple proof of the central limit theorem, which really is (or at least can be stated as) a result in Fourier analysis, and sketch the modern proof of the Hardy–Ramanujan and Erdős–Kac theorems. If time permits we mention other results in probabilistic number theory and their connections to other parts of mathematics.